Fixed-fixed beam - Couple moment Mo at any point Calculator
Fixed-Fixed Beam - Couple Moment \( M_0 \) at Any Point
A fixed-fixed beam is a beam that is rigidly supported at both ends, preventing rotation and translation. When a couple moment \( M_0 \) is applied at any point along the beam, it creates internal moments along the beam, but does not affect the shear force. The beam reacts to the moment by developing a distribution of bending moments and corresponding deflections.
Key Concepts
- Couple Moment \( M_0 \): A pair of equal and opposite forces whose lines of action do not coincide, creating a rotational effect but no net force. It is applied at a specific point on the beam, causing bending without altering shear forces.
- Fixed-Fixed Beam: A beam supported at both ends with fixed supports that prevent any displacement or rotation at the supports.
- Shear Force: The shear force remains unchanged by the couple moment because it is not associated with any net force, but it affects the bending moment distribution.
- Bending Moment: The bending moment is altered due to the application of the couple, which induces a moment that varies along the length of the beam.
- Deflection: The deflection is influenced by the magnitude and location of the applied couple moment, resulting in a curvature that is more pronounced near the center of the beam.
Behavior of the Fixed-Fixed Beam
- Reaction Forces:
- The couple moment applied at any point along the beam does not alter the reaction forces at the supports, since a couple has no net force. The reactions remain determined by the equilibrium of forces at the supports.
- Shear Force Diagram:
- The shear force remains constant along the length of the beam and is unaffected by the application of a couple moment.
- The shear force diagram is flat, as the couple moment does not induce a net force.
- Bending Moment Diagram:
- The bending moment diagram is affected by the couple moment and shows a discontinuity at the point where the couple is applied.
- At the point of application of the couple moment \( M_0 \), there is a sudden change in the bending moment, creating a jump in the diagram.
- The magnitude of the bending moment depends on the position of the applied couple and is typically symmetric for a couple applied at the center.
- Deflection: The deflection due to a couple moment can be calculated using standard beam deflection formulas. The maximum deflection typically occurs at the location of the couple or near the center of the beam. \[ \delta = \frac{M_0 L^2}{16 E I} \] where \( M_0 \) is the applied couple moment, \( L \) is the length of the beam, \( E \) is the modulus of elasticity, and \( I \) is the moment of inertia of the beam's cross-section.
Applications
- Structural Engineering: Often encountered in beams with applied torque or rotational loads, such as beams in machines or structures under twisting moments.
- Mechanical Systems: Found in mechanical systems where couple moments occur, such as rotating shafts or beams under torsional loading.
- Construction: Relevant in the analysis of beams subjected to moments applied at specific points, such as beams carrying equipment that applies rotational forces.
Formula
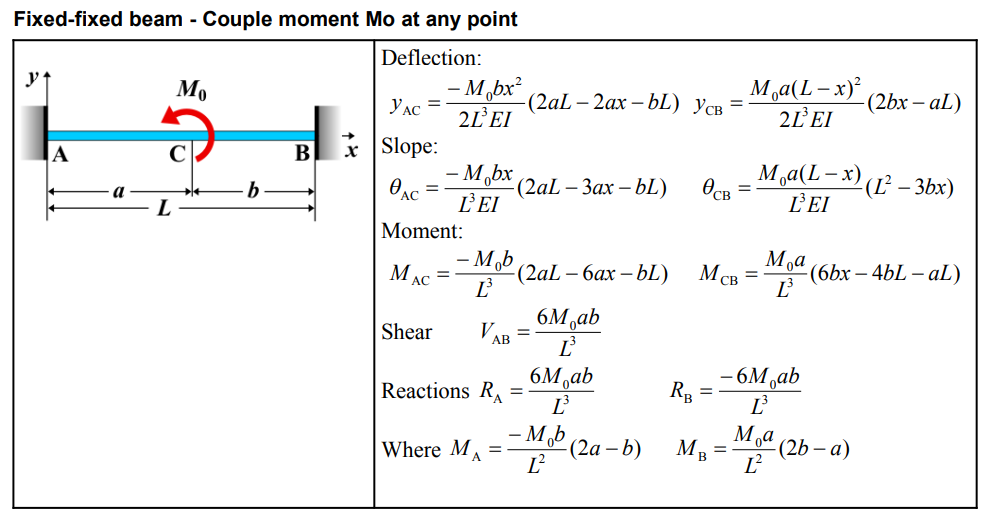
| Parameter | Formula |
|---|---|
| Deflection (AC) | \( y_{AC} = \frac{-M_0 b x^2}{2 L^3 EI} (2aL - 2ax - bL) \) |
| Deflection (CB) | \( y_{CB} = \frac{M_0 a (L - x)^2}{2 L^3 EI} (2bx - aL) \) |
| Slope (AC) | \( \theta_{AC} = \frac{-M_0 b x}{L^3 EI} (2aL - 3ax - bL) \) |
| Slope (CB) | \( \theta_{CB} = \frac{M_0 a (L - x)}{L^3 EI} (L^2 - 3bx) \) |
| Moment (AC) | \( M_{AC} = \frac{-M_0 b}{L^3} (2aL - 6ax - bL) \) |
| Moment (CB) | \( M_{CB} = \frac{M_0 a}{L^3} (6bx - 4bL - aL) \) |
| Shear | \( V_{AB} = \frac{6M_0 ab}{L^3} \) |
| Reactions (RA) | \( R_A = \frac{6M_0 ab}{L^3} \) |
| Reactions (RB) | \( R_B = \frac{-6M_0 ab}{L^3} \) |
| Where (MA) | \( M_A = \frac{-M_0 b}{L^2} (2a - b) \) |
| Where (MB) | \( M_B = \frac{M_0 a}{L^2} (2b - a) \) |
Definitions
| Symbol | Physical quantity | Units |
|---|---|---|
| E·I | Flexural rigidity | N·m², Pa·m⁴ |
| y | Deflection or deformation | m |
| θ | Slope, Angle of rotation | - |
| x | Distance from support (origin) | m |
| L | Length of beam (without overhang) | m |
| M | Moment, Bending moment, Couple moment applied | N·m |
| P | Concentrated load, Point load, Concentrated force | N |
| w | Distributed load, Load per unit length | N/m |
| R | Reaction load, reaction force | N |
| V | Shear force, shear | N |
 Home
Home Back
Back